
1000以上 P Qp2 Q2 P Qp2 Q2 ニスヌーピー 壁紙
For example there is no necessary connection between P='22=4' and Q='Washington is the capital of USA',still the inference P > Q is valid,that is always a TRUE implies a TRUE irrespective of the relations between the terms of the propositions P and Q in the russelian (material) definitionOn the other hand there is a necessary one between PP→Q means If P then Q ~R means NotR P ∧ Q means P and Q P ∨ Q means P or Q An argument is valid if the following conditional holds If all the premises are true, the conclusion must be true Some valid argument forms (1) 1 P 2 P→Q C Therefore, Q
If p.q=pq then angle
If p.q=pq then angle-J K CET 17 Let P and Q are matrices such that PQ = Q and QP = P , then P2 Q2 = (A) P (B) Q P Q (D) P Q Check Answer andLet p and q are two statements then "if p then q" is a compound statement, denoted by p→ q and referred as a conditional statement, or implication The implication p→ q is false only when p is true, and q is false;
If P Q Is Small Compared To Either P Or Q Then Show That N P Q N 1 P N 1 Q N 1 P N 1 Q Hence Find
Hypotheses followed by a conclusion is called an Ifthen statement or a conditional statement This is noted as p → q This is read if p then q A conditional statement is false if hypothesis is true and the conclusion is false The example above would be false if it said "if you get good grades then you will not get into a good college"P = {a, b, c} and Q = {r} P × Q = {a, b, c} × {r} P × Q = { Q × P = {r} × {a, b, c} Q × P = { (a, r), (b, r), (r, a), (r, b), Since (a, r) ≠ (r, a) P × Q ≠ Q × P Since the corresponding first and second elements are not equal, hence the two ordered pairs are not equalAnswer > \textbf{Q }\text{If }p, q, r\text{ and } s\text{ are in continued proportion, then }\\\frac{ps}{qr}\ge x \text{What is the value of }x\,?
If p is true and q is false, then p>q is never true So, the correct option is (c) 4 When p is false and q is true, then p or q is always true So, the correct option is (a) 5 If p>q is true and q is true, then p may be true or false So, the correct option is (b) Advertisement A rational number is a number which can be expressed in the form p/q where p and q are integers and p>0If p/q and r/s are two rational numbers then(p/q)*(r/s) = (p*r)/(q*s)You may need to check0 votes 1 answer If the pth and qth terms of a GP are q and p respectively, show that its (p q)th term is
If p.q=pq then angleのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In | If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In | 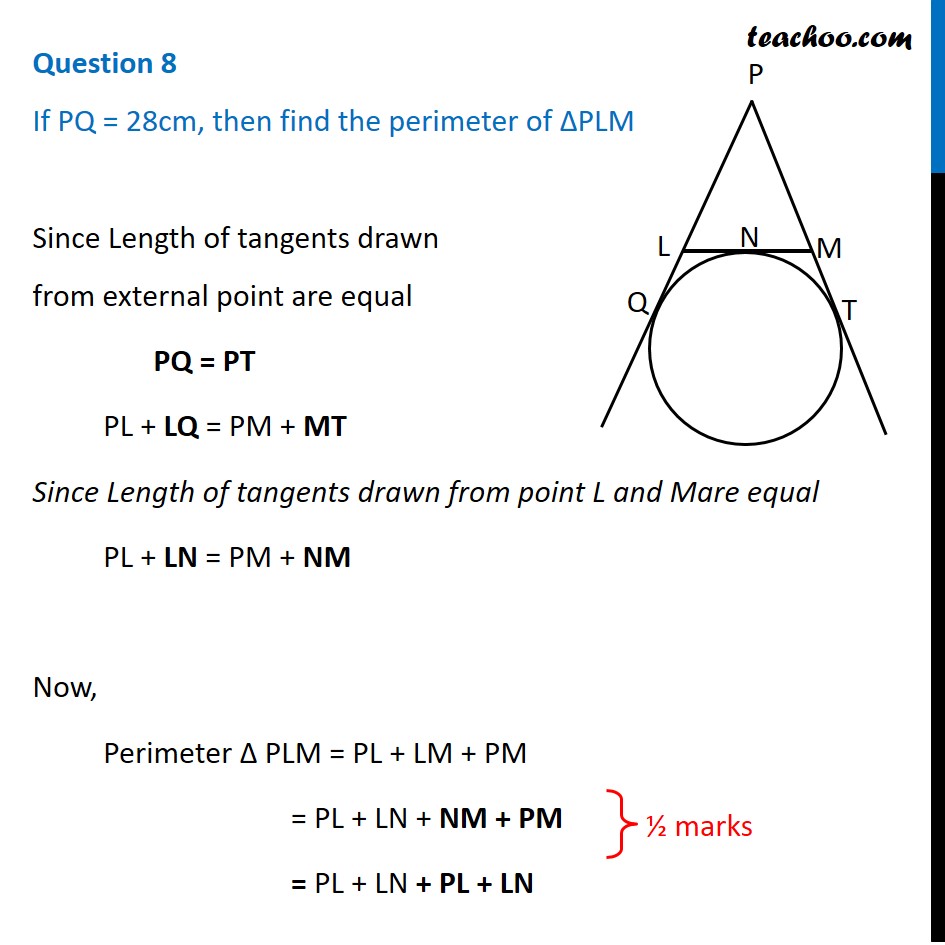 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In | 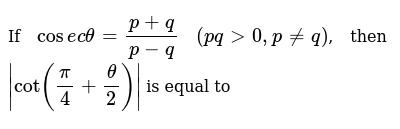 If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In | If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In | If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In | If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In | 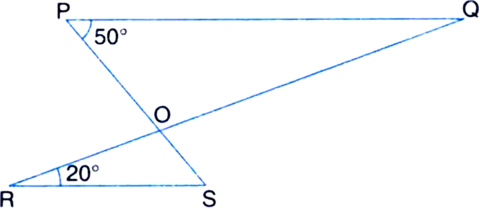 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In | 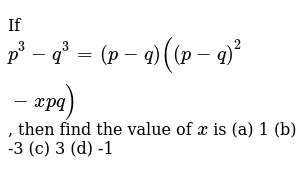 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In | 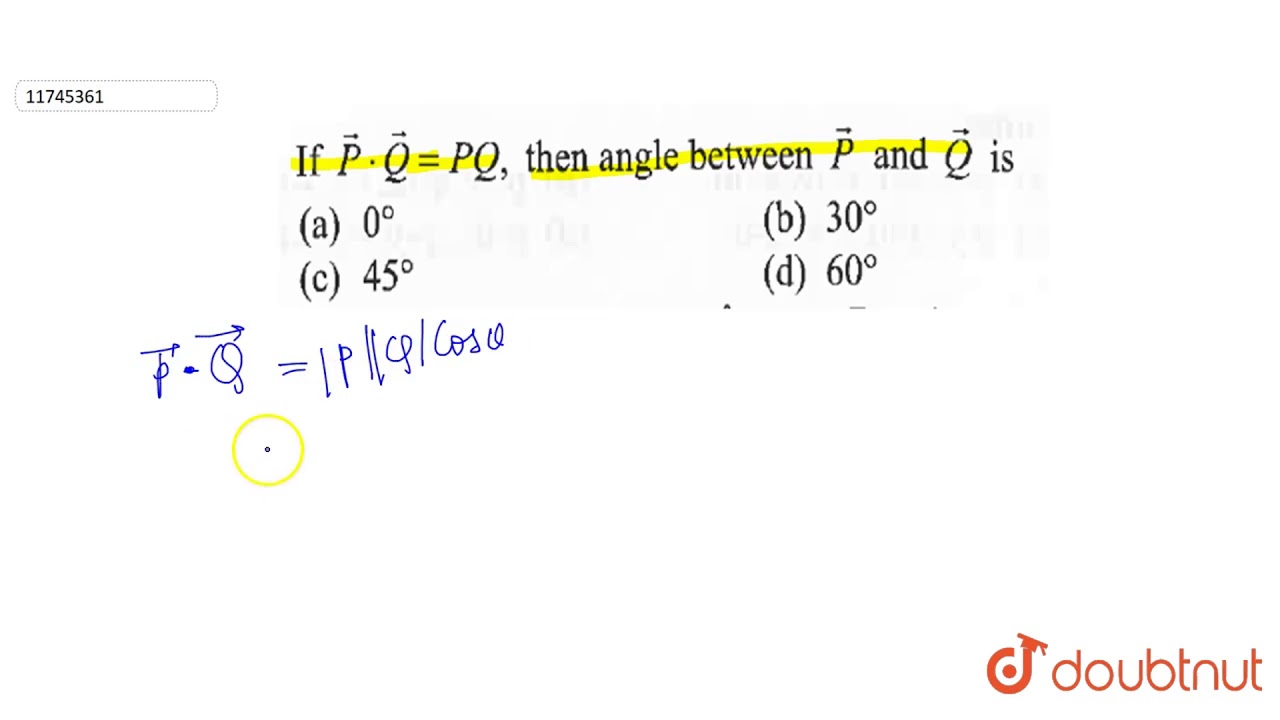 If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
 If P Q 1 Pq Then P Q Is Equal To Brainly In | If P Q 1 Pq Then P Q Is Equal To Brainly In |  If P Q 1 Pq Then P Q Is Equal To Brainly In |
If P Q 1 Pq Then P Q Is Equal To Brainly In |
If p times the pth term of an AP is q times the qth term, then what is (p q)th term equal to?The part of a conditional statement (If p, then q) introduced by the word then Deductive Argument An argument intended to provide logically conclusive support for its conclusion described as valid or invalid Denying the Antecedent An invalid argument form If p, then q Not p Therefore, not q
Incoming Term: if p+q=p-q then, if vector p+q=p-q then, if cosec theta=(p+q)/(p-q) then cot((pi)/(4)+(theta)/(2))=, if p/q+q/p=1 then p3+q3=, if cos p cos q p q then, if p.q=pq then angle, if p+q=r and p-q=s then r2+s2 is equal to, if p*q=2p+q then 2*3 is, if p + q equal to p minus q then, if p q p r then which is true,



